 Loading...
Loading...
Q.01
Solution:
![]()
From the graph, we see that
at
at
Instantaneous velocity at 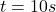 is equal to the slope of the tangent line at
is equal to the slope of the tangent line at 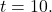
![]()
![]()
![]()
Q.02
Solution:
We shall find instantaneous velocity at From the graph, we see that
at
at
Instantaneous velocity at 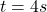 is equal to the slope of the tangent line at
is equal to the slope of the tangent line at 
at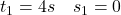
at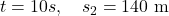
Instantaneous velocity at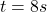 is equal to the slope of the tangent line at
is equal to the slope of the tangent line at 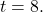
![]()
![]()
![]()
at
at
Instantaneous velocity at
![]()
![]()
![]()
Q.03 The accompanying figure shows the position versus time curve… (a) The average velocity over the interval 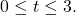 (b) The values of
(b) The values of  at which the instantaneous velocity is zero.
at which the instantaneous velocity is zero.
Solution:
From the graph, we have
at
at
![]()
![]()
We know that the points where the tangent line is horizontal are the points at which the slope of the tangent line is zero. ie the points where the instantaneous velocity is zero.
From the graph, we have that
![]()
![]()
![]()
![]()
![]()
Q.04 The accompanying figure shows the position version time curve…
Solution: