EXERCISE SOLUTION-0.1, Page#11, Calculus Book [10th Edition] by Howard ANTON, IRL BEVINS, and STEPHAN DAVIS. This section of the book consists of the definition of functions and their domain and range. The functions can be described by tables, graphs, formulas, and words. You can find the solutions to problems in this section which will help to get the concept of functions.
 Loading...
Loading...
Q.02
Solution:
(a) For  , and
, and  .
.
(b) There is no value of for which
for which  .
.
(c) For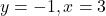 .
.
(d) For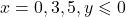 .
.
(e) maximum value of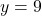 at
at 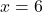 miniment value of
miniment value of 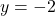 at
at  .
.
(b) There is no value of
(c) For
(d) For
(e) maximum value of
Q.03
Solution:
(a) It is a function because no vertical line intersects the graph more than once.
(b) It is a function because no vertical line intersects the graph more than once.
(c) It is not a function because the vertical line test fails.
(d) It is not a function because the vertical line test fails.
(c) It is not a function because the vertical line test fails.
(d) It is not a function because the vertical line test fails.
Q.04
Solution:
(a)
![]()
The function
The natural domain of ![]() consists of all real numbers ie
consists of all real numbers ie ![]() .
.
Natural domain of ![]()
Natural domain of ![]()
Natural domain of ![]() natural domain
natural domain ![]() if
if ![]() is excluded from the natural of
is excluded from the natural of ![]() . i.e.
. i.e. ![]() on
on ![]() .
.
(b)
![]()
The function
The function
Therefore the natural domain of
Q.07
Solution:
(a)
![]()
![Rendered by QuickLaTeX.com \[\begin{aligned}& f(0)=3(0)^2-2=-2 \\ \\& f(2)=3(+2)^2-2=10 \\ \\& f(-2)=3(-2)^2-2=10 \\ \\& f(3)=3(3)^2-2=25 \\ \\& f(\sqrt{2})=3(\sqrt{2})^2-2=4 \\ \\& f(3 t)=3(3 t)^2-2=27 t^2-2\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-f784d63cc525de14eb90368f2d766024_l3.png)
Q.07b
Solution:
![Rendered by QuickLaTeX.com \[\begin{aligned}& f(x)= \begin{cases}\frac{1}{x}, & x>3 \\ \\2 x & x \leq 3\end{cases} \\ \\& f(0)=2(0)=0 \\ \\& f(2)=2(2)=4 \\ \\& f(-2)=2(-2)=-4 \\ \\& f(3)=2(3)=6 \\ \\& f(\sqrt{2})=2 \sqrt{2}, \\ \\& f(3 t)=\left\{\begin{array}{l}\frac{1}{3 t} \quad 3 t>3\\ \\2(3 t) \quad 3 t \leq 3\end{array}\right. \\ \\& f(3 t)= \begin{cases}\frac{1}{3 t} & t > 1\\ \\6 t & t \leq 1\end{cases}\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-6a22993519ed049abaf9304f55dc27dd_l3.png)
Q.08a
Solution:
![]()
![]()
Q.08b
Solution:
![Rendered by QuickLaTeX.com \[\begin{aligned}g(x) & = \begin{cases}\sqrt{x+1} & x \geqslant 1 \\ \\3 & x<1\end{cases} \\ \\g\left(t^2-1\right) & = \begin{cases}\sqrt{t^2-1+1} & t^2-1 \geqslant 1 \\ \\3 & t^2-1<1\end{cases}\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-3e8d5db810fe72f4f96163afdb49c7f5_l3.png)
![Rendered by QuickLaTeX.com \[\begin{aligned}& g\left(t^2-1\right)= \begin{cases}\sqrt{t^2} & t^2 \geqslant 1+1 \\ \\3 & t^2<1+1\end{cases} \\ \\& g\left(t^2-1\right)= \begin{cases}|t|, & t^2 \geqslant 2 \\ \\3 & t^2<2\end{cases}\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-6dfce84d6e3062b56e167522e64f9144_l3.png)
Q.09 Find the natural domain and determine the range of each function.
Solution:
(d)
![Rendered by QuickLaTeX.com \[\begin{aligned}& G(x)=\sqrt{x^2-2 x+5} \\ \\& x^2-2 x+5=x^2-2 x+1+4 \\ \\& =(x-1)^2+4 \geqslant 4 \\ \\& G(x)=\sqrt{x^2-2 x+5}=\sqrt{(x-1)^2+4} \geqslant \sqrt{4}=2 \\ \\& \Rightarrow G(x) \geq 2 \quad \forall x \\ \\&\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-2fc2322f8f047d64da3c24e822f6cb7c_l3.png)
Range
Q.09(e)
Solution:
![Rendered by QuickLaTeX.com \[</div> <div> \begin{aligned}& h(x)=\frac{1}{1-\sin x} \\ \\& h(x) \text { is defined for those} x \text { for which } 1-\sin x \neq 0 \\ \\ & \sin x \neq 1 \Rightarrow x \neq \sin ^{-1}(1) \\ \\ & \Rightarrow x \neq \text { all odd multiple of } \frac{\pi}{2} \\ \\ & \Rightarrow \quad x \neq\left(2 n+\frac{1}{2}\right) \pi, \quad n=0, \pm 1 \pm 2, \ldots \\ \\& \Rightarrow \quad -1\leq \sin x < 1 \quad \forall \quad x \neq\left(2 n+\frac{1}{2}\right) \pi, \quad n=0, \pm 1 \pm 2, \ldots\\ \end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-cb813b1ed0e56eb4cbd9bdcb81a663c9_l3.png)
Multiplying by ![]()
![Rendered by QuickLaTeX.com \[\begin{aligned} & \Rightarrow \quad -1<-\sin x \leq 1 \\ \\ & \Rightarrow \quad -1+1<-\sin x+1 \leq 1+1 \\ \\ & \Rightarrow \quad 0<-\sin x+1 \leq 2 \\ \\ & \Rightarrow \quad -\sin x+1 \leq 2 \\ \\ & \Rightarrow \quad \frac{1}{1-\sin x} \geq \frac{1}{2} \\ \\& \Rightarrow \quad h(x) \geqslant \frac{1}{2} \\ \\& \therefore \quad \text { Range: } y \geq \frac{1}{2} .\end{aligned}\]](https://genesismath.com/wp-content/ql-cache/quicklatex.com-c5903ece536edd5da0b1d7aa673178c5_l3.png)